WELFARE ECONOMICS
Satiation in the Solow Model
David Hudgins, Department of Economics University of Oklahoma 1/1/2011 4:46:39 AM
 Modern economic growth theory began with Solow’s (1956) paper, A Contribution to the Theory of Economic Growth, which developed the standard neoclassical growth model. Mankiw, Romer, and Weil (1992) augmented and empirically tested the Solow model in their paper, A Contribution to the Empirics of Economic Growth. The goal of this analysis is to complete the trilogy with a contribution to the purpose of economic growth by developing an index that defines and evaluates economic growth when nations are characterized by satiation. The biggest problem with the neoclassical Solow growth model is addressed only by Schumacher (1973). This problem is that growth in capital and the wrong types of technology actually diminishes the quality of both human life and of nature. The Solow model incorrectly defines economic growth and it offers absolutely no reason as to why it would be desirable.
Modern economic growth theory began with Solow’s (1956) paper, A Contribution to the Theory of Economic Growth, which developed the standard neoclassical growth model. Mankiw, Romer, and Weil (1992) augmented and empirically tested the Solow model in their paper, A Contribution to the Empirics of Economic Growth. The goal of this analysis is to complete the trilogy with a contribution to the purpose of economic growth by developing an index that defines and evaluates economic growth when nations are characterized by satiation. The biggest problem with the neoclassical Solow growth model is addressed only by Schumacher (1973). This problem is that growth in capital and the wrong types of technology actually diminishes the quality of both human life and of nature. The Solow model incorrectly defines economic growth and it offers absolutely no reason as to why it would be desirable.
The Schumacher Index for Consumers and Workers
The purpose of economic growth is to improve the total life experience of the individual participants. Based on this objective, the only true definition of economic growth becomes clear. Economic growth is an improvement in the overall welfare of the participants of an economy through the allocation and uses of its economic resources. Economic growth does not mean the growth of the total consumption of goods or the growth of income. This can be stated directly in equation (1), where ^ represents the new Schumacher index of life experience for a representative individual and Lambda is the instantaneous life experience at any given moment of time t.
 ---------(1)
---------(1)
The instantaneous life experience is function of the person’s consumption welfare index (CWI) of material output, the leisure welfare index (LWI), and the level of the personal development index (D).
The Solow model defines the economic growth rate to be the growth rate in per capita income, which creates two problems. Firstly, higher income growth is not synonymous with higher growth in total personal welfare; thus, economic growth is incorrectly defined. Secondly, the model does not state any purpose for having a higher or lower growth rate. The Ramsey model, as well as other standard models, fills in this gap by defining consumer welfare with a utility function that is strictly increasing in total consumption (Romer, 2005). This approach has permeated the field of economics. In these models, consumers maximize their welfare by maximizing present and discounted future consumption.
Schumacher (1973, p. 54) recognized this basic problem with the model and stated that “the aim should be to obtain the maximum of human well-being with the minimum of consumption.” He advocated a pragmatic, non-quantitative approach that completely abandoned the neoclassical growth model. However, the Solow model still offers much promise, as long as it is placed on a firm foundation that has purpose and applicability. The model needs to be re-formulated utilizing the above specifications regarding the human welfare resulting from consumption. A consumption welfare index (CWI) that characterizes this is the following modified gamma function, where C denotes an individual’s consumption of output.
 ---------(2)
---------(2)
 Figure 1 shows a graph of this function where Q1 = 0.026722, Q2 = 20, and Q3 = 0.2. The value of CWI will be 0 when consumption is 0, since an individual must consume some amount of output to live. It will reach a maximum satiation point, or bliss point, once consumption reaches a level that is above subsistence and just large enough to allow the individual to give the maximum focus toward meaningful pursuits.
Figure 1 shows a graph of this function where Q1 = 0.026722, Q2 = 20, and Q3 = 0.2. The value of CWI will be 0 when consumption is 0, since an individual must consume some amount of output to live. It will reach a maximum satiation point, or bliss point, once consumption reaches a level that is above subsistence and just large enough to allow the individual to give the maximum focus toward meaningful pursuits.
The term Q1 can be chosen so that the maximum value of the index is 1, as in this example. The function will decline and eventually approach Q1 > 0 as consumption increases beyond the optimal value, and will equal Q1 in the limit as consumption approaches infinity. This reflects human behavior where excessive attachment to hedonistic consumption actually detracts from the quality of life.
Satiated preferences are essential to modeling growth. Economic theory has typically assumed monotonic preferences where hedonistic consumers always prefer more to less, and has viewed satiation as a theoretical anomaly with little practical use. Varian (2010, p. 45) even stated that “Economics would not be a very interesting subject in a world where everyone was satiated in their consumption of every good.” This view is misguided.
The interest of economics is to provide guidance for individuals to find balance between the bliss point combination of consumption and abstention for all goods. Due to the negative environmental and health effects of 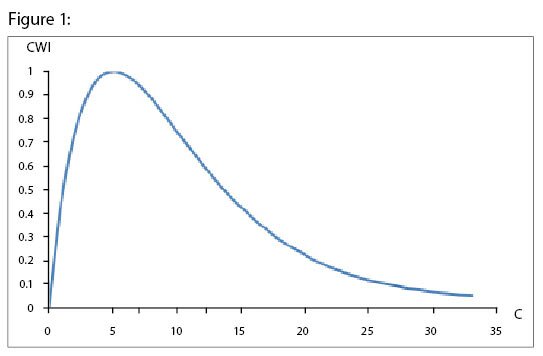
overproduction, it could be argued that the U.S. is currently producing and consuming beyond its bliss point level. For example, ten states in the U.S. currently have an obesity rate of over 29 percent (Robert Wood Johnson Annual Report, 2010). This shows evidence that welfare would be enhanced with a smaller per capita consumption. Although this is only one brief summary example, the application to India and other developing nations is obvious. Any development agenda that is based upon measuring progress by Western idealized consumption maximization is doomed to lead to immiserizing growth, rather than generating true economic and social progress.
The neoclassical growth model also ignores leisure. After their basic material needs are met, consumers will place an increasing value on leisure and smaller value on consumption. Although this backward-bending labor supply function appears in some models, it is absent from the Solow model.
The term leisure needs to be redefined. It does not mean that an individual is only gaining leisure-derived welfare while resting and doing nothing. It is incorrect to view a worker who is enjoying leisure, and not working for a given unit of time, as not being productive. On the contrary, it means that an individual is equally productive when allocating time to leisure, because productivity is defined as the amount life experience that the individual gains per unit of time, where the objective is to maximize this experience. Leisure does not equal rest in this usage; rather, it is defined as any time not spent during the production process of economic goods.
The leisure welfare index (LWI) is not the inverse of the time spent on labor, however. Schumacher (1973) points out that each individual can be assumed to gain some satisfaction from providing labor in the production of the economic output. This labor actually enhances an individual’s welfare by providing a complementary experience up to a certain level of hours. The optimal labor-to-leisure ratio is not zero, although it is presumably less than one. Otherwise, consumers would prefer to spend more hours of a day working than pursuing leisure.
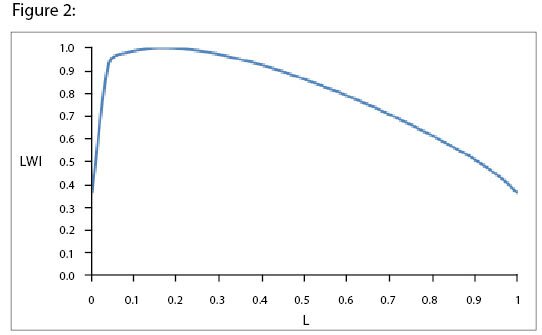
Let L represent the percentage of that time spent on working to produce economic output, so that (1 – L) represents the percentage of that time spent on leisure. A leisure welfare index, LWI, that captures a person’s preferences can be expressed as follows.
 ---------(3)
---------(3)
The maximum value for the LWI index will occur at LWI(v) = 1. Presumably, v < 1/3, since a person would optimally work less than an eight-hour workday (1/3 of a 24-hour day). Figure 2 shows the index values where v = 1/6, where individuals maximize their welfare by working an average of four hours out of every 24-hour day.
How much of economic growth actually represents improvement in life experience? For Schumacher, this was the primary question. What the previous models essentially treat as progress is almost the antithesis of development. When a lesser developed country becomes more attached to consumption, and substitutes its citizens’ focus from personal goals toward pure consumption, this actually represents reduced life experience, rather than its enhancement. Increased income does not equal progress, and the standard by which the model measures consumer welfare must account for this.
The final input into the consumer welfare function is the personal development index, D, which is given by the following expression.
 -----------(4)
-----------(4)
It is a measure of the quality of individual experiences. This will depend in part on the individual’s cumulative level of education and training, which is denoted by H, and in part on the combination of physical consumption and leisure to produce levels of life experience, which form a multiplicative interaction. The H variable could be measured by various schemes, but higher values represent a higher quality and quantity of training, and could also include a measure at the aggregated country level that increases with greater physical and emotional fitness.
This Schumacher-Solow index discussed here tacitly addresses the environmental dilemma. Since the individual and collective national welfare function penalizes consumption that occurs beyond the level that maximizes life experience, the use of resources will be held in check. As long as the optimal value for resource usage is less than the rate of regeneration for renewable resources, the entire system will be sustainable.
Each of the three indices above can now be used in equation (1) to form the full Schumacher objective function, where 0 ≤ CWI, LWI ≤ 1. If the functional form of Q is Cobb-Douglas, then the objective function in equation (1) becomes
 ----------(5)
----------(5)
Substituting equations (2), (3), and (4) into (5) yields the following, where life experience is a function of labor (L), consumption (C), and the education and health index (H).
.jpg)
-------(6)
Equation (6) can also be used for the aggregate economy by using the variables consumption per human contribution unit (c) and education per human contribution unit (h), instead of consumption (C) and education (H).
Satiation must be included in population growth. Labor growth in the neoclassical model creates two major problems. Firstly, the growth in the labor force is not exogenous, since the decisions regarding reproduction and labor force participation are determined by the level of development and income. More importantly, there is no penalty in the Solow model for continuous population growth. A common problem with developing countries on a finite globe is excessive population growth.
The labor force index N can be written analogously to figure 1 and equation (2), where P is the total population.
 --------(7)
--------(7)
In this case, (L P) is the amount of labor that is supplied, and N is the contribution which that labor supply makes toward output production. Through this labor index, output itself has a built-in quality of life component since it is a function of N, rather than just total labor hours employed. Given a labor force participation rate of L, then all else constant, the shape of the graph would be similar to that in Figure 1, where the labor index N has an interior maximum. In this model, once the labor supply equals its optimal value, the optimal growth rate will be n = 0.
This recognizes that the economy is not an open system, and addresses environmental concerns by limiting the population size and the output produced. It also helps to explain a crucial empirical failing of the Solow model, which suggests that highly skilled workers will move from developed countries to developing countries. Countries with populations that exceed their optimum levels will be characterized by a lower quality of life. Therefore, the most skilled individuals will migrate toward the developed countries to improve their own life experience.
Conclusion
This Solow-Schumacher Growth Model has purpose and direction. Although these two individuals originally approached growth from seemingly dissonant perspectives, the proper synthesis of these two modes of analysis has indeed struck a  resonant chord. The revised model allows nations to optimize consumption, rather than maximize it. Further research should empirically explore the desired target values and actual behavior. Each economy’s current average workweek, population, saving rates, education level, capital stock, and production levels should be assessed relative to the bliss point values that maximize the life experience. If a country’s average workweek is 42 hours, and the optimal workweek is 28 hours, and that the levels of economic production are 33% higher than the bliss level, then public policy should give incentives for decreases in hours worked, production, and per capita income. This would likely be the case for most “developed” economies.
resonant chord. The revised model allows nations to optimize consumption, rather than maximize it. Further research should empirically explore the desired target values and actual behavior. Each economy’s current average workweek, population, saving rates, education level, capital stock, and production levels should be assessed relative to the bliss point values that maximize the life experience. If a country’s average workweek is 42 hours, and the optimal workweek is 28 hours, and that the levels of economic production are 33% higher than the bliss level, then public policy should give incentives for decreases in hours worked, production, and per capita income. This would likely be the case for most “developed” economies.
Schumacher (1973) observed that there seems to be an inverse relationship between countries’ real leisure time and the amount of machinery and wealth at its disposal. The whole concept of “developed” countries needs to be redefined. A country that is on either side of the optimal bliss point based on its per capita consumption, or leisure time, is “less-developed” than a country that is closer to the bliss point. Development in this context is no longer a linear progression of income, but rather a movement toward an optimal balance of the factors discussed above. Some of the “less-developed” countries under the current classification would become re-classified as being the more developed countries if their overall life-experience is rated highly, in spite of considerably lower per capita income, consumption, education, life expectancy, and other current benchmarks. The Human Development Index, for example, embeds the assumption that welfare is strictly increasing with GDP and life expectancy. The Schumacher index is superior since it considers quality as well as quantity and satiation.
References and Additional Thinking
Easterly, W., 2002. The elusive quest for growth: economists’ adventures and misadventures in the tropics (MIT Press, Cambridge, MA).
Mankiw N. G., Romer, D., Weil, D. N., 1992. A contribution to the empirics of economic growth. Quarterly Journal of Economics 107, 407 - 437.
Robert Wood Johnson Foundation, 2010. F as in fat - how obesity threatens america’s future. Annual Report,
http://www.rwjf.org/files/research/20100629fasinfatmainreport.pdf.
Romer, D., 2005. Advanced macroeconomics, 3rd ed., (McGraw-Hill , New York, NY).
Schumacher, E.F., 1973. Small is beautiful: economics as if people mattered, (Harper & Row, New York, NY).
Solow, R. M., 1956. A contribution to the theory of economic growth. Quarterly Journal of Economics 70, 65-94.
Varian, H.R., 2010. Intermediate microeconomics: a modern approach, 8th ed. (Norton, New York, NY).
(David Hudgins holds a B.S. degree in Business Administration from Oklahoma State University, where he received the Richard H. Leftwich Award in Economics in 1988. He received an M.S. and Ph.D. degree in economics from the University of Illinois at Urbana-Champaign in 1993. He is currently a professor at the University of Oklahoma, where he teaches both graduate and undergraduate courses in economics and statistics. He has published research in various books and journals on various topics in international and applied economics.
The views expressed in the write-up are personal and do not re?ect the official policy or position of the organization.)
<
|
| |
|
| |
|
|
*
|
| Name: |
* |
| Place: |
* |
| Email: |
* *
|
| Display Email: |
|
| |
|
| Enter Image Text: |
|
| |
|
